|

Self-trapping of the
lowest singlet exciton in the center (shown) of a fluorene dodecamer in
the first excited state equilibrium geometry.
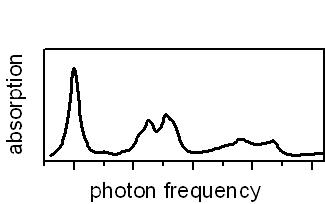
Low-temperature linear absorption of a fluorene octamer calculated from
density-functional theory based ground and excited state information
including electronic and vibrational degrees of freedom.
|
|
Great
strides have been made over the last ten years in realising the
enormous potential of organic semiconductor materials in that they are
cheap, flexible and easily processed. The development of early polymer
amplifiers and lasers today is following a similar trajectory to that
of inorganic optoelectronic devices in the 1980's.
One key aspect in advancing polymer photonic and optoelectronic devices
further, is the development of a profound theoretical understanding of
the nonlinear optical excitation dynamics in organic semiconductor
materials in the relevant excitation regimes. In describing and
modelling inorganic semiconductor optoelectronic devices, such as
amplifiers and lasers, equation of motion or density matrix theory
approaches (the so-called Semiconductor Bloch Equations) have proven
extremely successful. For organics systems, gaining a similarly high
level of theoretical understanding as for their inorganic counterparts
would be most desirable.
Typical organic materials of interest (e.g., polyfluorenes and fluorene
based co-polymers) show optical properties that are, even in
technologically relevant solid-state systems, essentially molecular in
nature. Strong coupling of electronic and nuclear degrees of freedom in
these systems gives rise to a vibronic substructure of molecular energy
levels which is a fundamental ingredient in typical organics-based
all-optical amplification and lasing schemes, and which is in strong
contrast to inorganic semiconductor systems. On the other hand,
important analogies with inorganic semiconductor optoelectronic systems
exist, such as, e.g., the importance of excitonic effects, albeit with
much higher binding energies, and the importance of exciton-exciton
scattering processes at elevated excitation densities.
To model the optical response of a given system requires knowledge not
only of the population of the system's excitations but also of the
induced polarisations, as these are the sources for the propagating
light field. To be able to meet these requirements, in our present
project we use and develop a theoretical approach that combines the
strength of (i) density-functional theory (DFT) calculations for
molecular systems and (ii) a density-matrix theory based many-particle
approach. The DFT calculations give detailed ab-initio insight into
ground and excited vibronic state properties on the molecular level.
This paves the way to formulate a microscopically founded model
Hamiltonian describing a molecular solid-state system. Starting from
this Hamiltonian, density matrix equations of motion are derived to
calculate (non-perturbatively in the external light field) the nolinear
optical excitation dynamics of the system. Within this theoretical
framework, important many-particle aspects such as inter-molecular
excitation transfer and scattering, and relaxation and dephasing of
vibronic excitations can systematically be included and studied. Also,
direct inclusion of the propagation light field in the description
allows to study important radiative effects such as amplified
spontaneous emission (ASE) that is known to drastically influence
device performance at high excitation densities.
Our research is carried out in close collaboration with Ifor Samuel at
the University of St. Andrews, Organic Semiconductor Optoelectronics
Group
|